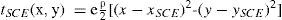Our goal was to examine the accuracy of metrics, calculated using a numerical eye model including the measurement of the monochromatic aberrations of the eye, to predict the contrast sensitivity (CS) and visual acuity (VA) visual benefits (VB) of correcting higher-order aberrations (HO).
MethodsWe measured, on the right eyes of 25 subjects (10 myopes and 15 emmetropes) aged 21 to 56 years, the 16 c/deg CS and high-contrast VA in two conditions of aberration corrections: (i) when correcting only the defocus and astigmatism terms and (ii) when dynamically correcting all the monochromatic aberration terms up to the 5th order. The measured VB was defined as the ratio of the performances between these two conditions of aberration corrections.
ResultsWe measured a VB of 1.25 and 1.64 respectively in term of VA and CS. We did not find any influence of age on the VB and no statistical significant difference between the myopic and emmetropic group. The contrast sensitivity VB was well correlated (r2=0.79) with the ratio of the modulation transfer functions calculated at 16 c/deg in both conditions of aberrations corrections (i.e. MTF16c/deg HO/MTF16c/deg SC). The levels of correlation between various metrics and measured visual acuity VB were lower (r2=0.30 in the better case), however the averaged VB was correctly predicted by the ratio of the intersections between the MTF and a typically neural contrast threshold function.
ConclusionsMetrics based on wave aberration measurements are able to predict the impact of monochromatic aberrations on CS.
Nuestro objetivo era analizar la validez y la precisión de los descriptores numéricos objetivos (calculados a partir de un modelo de ojo existente al que incorporamos las aberraciones monocromáticas del ojo de cada sujeto) a la hora de predecir los beneficios visuales (BV) sobre la sensibilidad al contraste (SC) o sobre la agudeza visual (AV) obtenidos tras corregir las aberraciones de alto orden.
MétodosMedimos la SC a 16 c/grado y la AV de alto contraste en el ojo derecho de 25 sujetos (10 miopes y 15 emétropes) de edades comprendidas entre los 21 y los 56 años. Las medidas se realizaron en dos condiciones distintas de corrección de aberraciones: (i) cuando sólo los términos de desenfoque y de astigmatismo estaban corregidos y (ii) cuando todas las aberraciones monocromáticas hasta 5° orden fueron corregidas dinámicamente.
La medida de los BV se definió como la razón de los resultados obtenidos en cada una de estas dos condiciones de corrección de aberraciones.
ResultadosLos BV sobre la AV y la SC fueron, respectivamente, de 1,25 y de 1,64. No encontramos ninguna dependencia de los BV con la edad del sujeto, ni ninguna diferencia estadísticamente significativa entre el grupo miope y el emétrope. Se obtuvo una notable correlación (r2=0,79) entre los BV sobre la sensibilidad al contraste y la razón de los valores de la función de transferencia de modulación (MTF) para 16 c/grado en sendos casos de corrección de aberraciones (es decir, el valor de MTF16c/grado AltoOrden / MTF16c/grado Seg.Orden). El grado de correlación entre diversos descriptores objetivos y los BV sobre la agudeza visual fue menor (r2=0,30 en el mejor de los casos). No obstante, el valor promedio de los BV se pudo predecir con bastante exactitud partiendo del punto de corte de la MTF (para una condición de corrección dada) con una función de contraste umbral neural típica, y calculando luego la razón de estos valores correspondientes a las dos condiciones de corrección analizadas.
ConclusionesLos descriptores numéricos basados en las medidas de la aberración de onda son capaces de predecir el impacto de las aberraciones monocromáticas sobre la SC.
It is well known that the human eye suffers from monochromatic aberrations in addition to defocus and astigmatism and that these aberrations degrade the retinal image and consequently limit the visual performance1. Adaptive optics (AO), recently used in the study of the human eye, enables the measurement and the correction of optical aberrations, thus permitting the evaluation of the visual benefit (VB) of correcting higher-order aberrations.
Previous measurements of the impact of the correction of higher-order aberrations on contrast sensitivity and visual acuity have been performed. In terms of contrast sensitivity, Liang et al.2 measured a 6-fold improvement at 27.5 c/deg on 2 subjects, across a 6mm pupil and in monochromatic light, whereas, Yoon and Williams3 measured at 16 c/deg, on 2 subjects and with the same pupil size, an improvement of contrast sensitivity by a factor of 1.9 when only monochromatic aberrations were corrected in polychromatic light. Yoon et al. attempted to predict the VB by calculating the ratio of MTFs computed with and without HO aberrations, they found at 16 c/deg a theoretical polychromatic VB of 2.8, which is quite different from their 1.9 measured VB. In terms of visual acuity, Yoon and Williams3 reported, on 7 subjects across a 6 mm pupil, a 1.2-fold and a 1.4-fold improvement after correcting the monochromatic aberrations in polychromatic light respectively at 575 Td (Troland, unit of retinal illuminance) and 57 Td. Poonja et al.4 measured on 6 subjects, using an Adaptive Optics Scanning Laser Ophtalmoscope (AOSLO) (e.g., in monochromatic light) and a pupil diameter of 5.89mm, a VB of 1.5 with an averaged visual acuity of 0.85 and 0.57 respectively before and after correction of the monochromatic aberrations.
Customized contact lenses, intraocular lenses and refractive surgery are now being developed to correct higher-order aberrations. The benefit from AO correction depends on the pupil size and the initial higher-order aberrations present in the eye. To address this issue, Guirao at al.5 calculated the visual benefit of correcting the monochromatic aberrations of 218 normal eyes and found great variation among eyes with some normal eyes showing almost no benefit and others a benefit higher than 4 at 16 c/deg across a 5.7 mm pupil, the average VB being 2.4.
The visual benefit should also depend on the ametropia6 since cortical and/or retinal factors are involved in the AO-corrected limit of resolution. Rossi et al.6 measured the VA using an AOSLO, in 10 low myopes and 9 emmetropes, the impact of correcting monochromatic aberrations. The myopic group improved from a mean of 0.83’ in the non-AO condition to a mean of 0.61’ with AO (e.g. a VB of 1.37). The emmetropes improved from a mean of 0.8’ without AO to a mean of 0.49’ with AO (e.g. a VB of 1. 64). Retinal and/or cortical factors could have limited the VA in low myopes after AO correction resulting in a lower VB in this population.
Recent studies7,8 have suggested that we might be adapted to our retinal image, which would have an impact on the visual benefit of correcting the eye's aberrations. Artal et al.7 wondered whether the visual system was adapted to the retinal image of its own eye; in other words, is the best overall subjective image quality obtained when a perfect retinal image is presented on the retina or when the retinal image is blurred by the aberrations of the eye's optics, indicating that the neural system is adapted to the particular pattern of its own eyes, and is able to compensate for the effects of this blur. Theirs results support the hypothesis of a neural compensation for the aberrations. Moreover, several studies9-12 demonstrated improvements in visual acuity after a period during which the subjects had been blurred by a defocus. Mon-Williams et al.9 measured, on 15 emmetropic subjects, an average visual acuity increase of 0.1 logMAR after a 30min wearing period of a +1.00 D defocus. George and Rosenfield10 found, on 13 emmetropic and 18 myopic subjects, an average visual acuity increase of 0.2 logMAR after a 2hours wearing period of a +2.50 D defocus. Rosenfield et al.11 measured on 22 slightly myopic subjects an increase of visual acuity of 0.23 logMAR after 3 hours without compensation. Cufflin et al.12 found after blur adaptation of 45 minutes, on 11 emmetropes and 11 early-onset myopes, an improvement in visual acuity of 0.17 logMAR and 0.23 logMAR following adaptation to +1 D and +3 D of defocus respectively. They also noted that acuity changes became significant after 30 minutes of exposure to optical defocus. These experiments show the effect of neural adaptation to a blurred retinal image on objective visual performance as well as on subjective responses. On the contrary, Pesudovs13 did not find any evidence for an adaptation to surgically induced blur until ten weeks after LASIK. In conclusion, these experiments showed that visual acuity improved following a period of adaptation to blur and, consequently, that the optics alone could not explain the changes in performances without assuming that some neural adjustments were interfering with the optics. In this case, a metric derived from wave aberrations measurements might not be able to predict the visual benefit of correcting the higher-order aberrations. However, it is not evident that the situation will be similar with another visual task such as contrast sensitivity.
Previous authors14-18 studied the link between metrics of image quality and visual performances or subjective image quality. The first objective was to determine the accuracy and precision of metrics for predicting the results of conventional sphero-cylindrical refraction from wavefront aberrations14,18. Guirao et al.14 found that image-plane metrics such as the volume under the MTF between 0 and 60 c/deg were able to predict the subjective refraction of the eye. The mean error between predicted and subjective refraction was about 0.1±0.08 D averaged across 6 eyes of 6 normal subjects whereas their five calculated image-plane metrics did not differ by more than 0.03 D. Similarly, Thibos et al.18 compared 33 metrics calculations to subjective refractions performed to the nearest 0.25 D on 200 normal and healthy eyes from 100 subjects. All the mean predicted values varied from −0.50 D to +0.25 D. The variability between various metrics was always lower than the ± 0.75 D test-retest variability in the measurement of refractive error19,20, meaning that the accuracy of metrics to predict subjective image quality should not be assessed this way. Marsack et al.16 used the previous data set to investigate the ability of 31 metrics derived from wave aberrations maps to predict changes in high-contrast logMAR acuity. The visual acuity loss were measured on simulated aberrated charts generated with a commercially available program called CTView© that introduces the aberrations into the charts by directly setting the wavefront Zernike coefficient values and performing a convolution of the resulting point-spread function with an image of an acuity chart. The visual strehl ratio computed in the frequency domain was found to be well correlated (r2=0.81) to the letters lost. However, the comparison between simulated letters and metrics - both calculated using the same input (e.g. the measured wavefront map) - is also questionable. A more rigorous experiment would have compared metrics and measured visual performances in real conditions by using real optics.
Chen et al.15 used matching blur experiment to test the accuracy of various metrics to predict the subjective quality of vision. The subjects had to compare the blur caused by a single aberration to a combination of aberrations. However, as mentioned by the author, blur is not a unity perceptual experience, meaning that the appearance of the blur is different as a function of the origin of the blur. In other words, it appears very difficult to adjust the level of the defocus blur to equalize the blur caused by a LASIK wave aberration, for example.
In order to examine the accuracy of metrics - calculated using a previously published numerical eye model21 including the measurement of the monochromatic aberrations of the eye - and to predict the visual benefit of correcting high-order aberrations, we measured visual performances before and after AO-correction.
MethodsGeneral MethodThe visual performances, 16 c/deg contrast sensitivities and high-contrast visual acuities, were randomly measured in two conditions of aberration correction: (i) when correcting only the defocus and astigmatism terms and (ii) when correcting all the monochromatic aberration terms up to the 5th order. The measured visual benefit in term of contrast sensitivity was defined as the ratio between the sphero-cylindrical-corrected contrast threshold and the 5th-order-aberration-corrected contrast threshold. Similarly, the visual acuity benefit was the ratio between the 2nd-order-aberration-corrected MAR and the 5th-order-aberration-corrected MAR. Visual performances measurements were made on the right eyes of 25 subjects aged 21 to 56 years (mean age: 38 years) with clear intraocular media and a pupil diameter larger than 5.5 mm under our testing conditions and without known pathology. Subjects had spherical refractive errors between -4 D and +1 D [spherical equivalent (M) =-0.97 D, standard error of mean (SEM) = 0.31 D] and astigmatism lower than 1.5 D (M=-0.28 D, SEM=0.10 D). Fifteen emmetropes and ten myopes were included. During the measurement, the subject's head was stabilized with a bite bar. We measured the eye's wave aberration in three conditions: (i) while the deformable mirror was set to an aberration-free shape corresponding to the eyes’ wavefront alone, (ii) while the deformable mirror was reshaped to correct the subject's astigmatism and (iii) while the deformable mirror was set to compensate for the aberrations up to the 5th order. Informed consent was obtained from each subject according to the tenets of the Declaration of Helsinki. The complete process of measurements took around 1 hour per subject and was realized in one session including several rest periods between measurements.
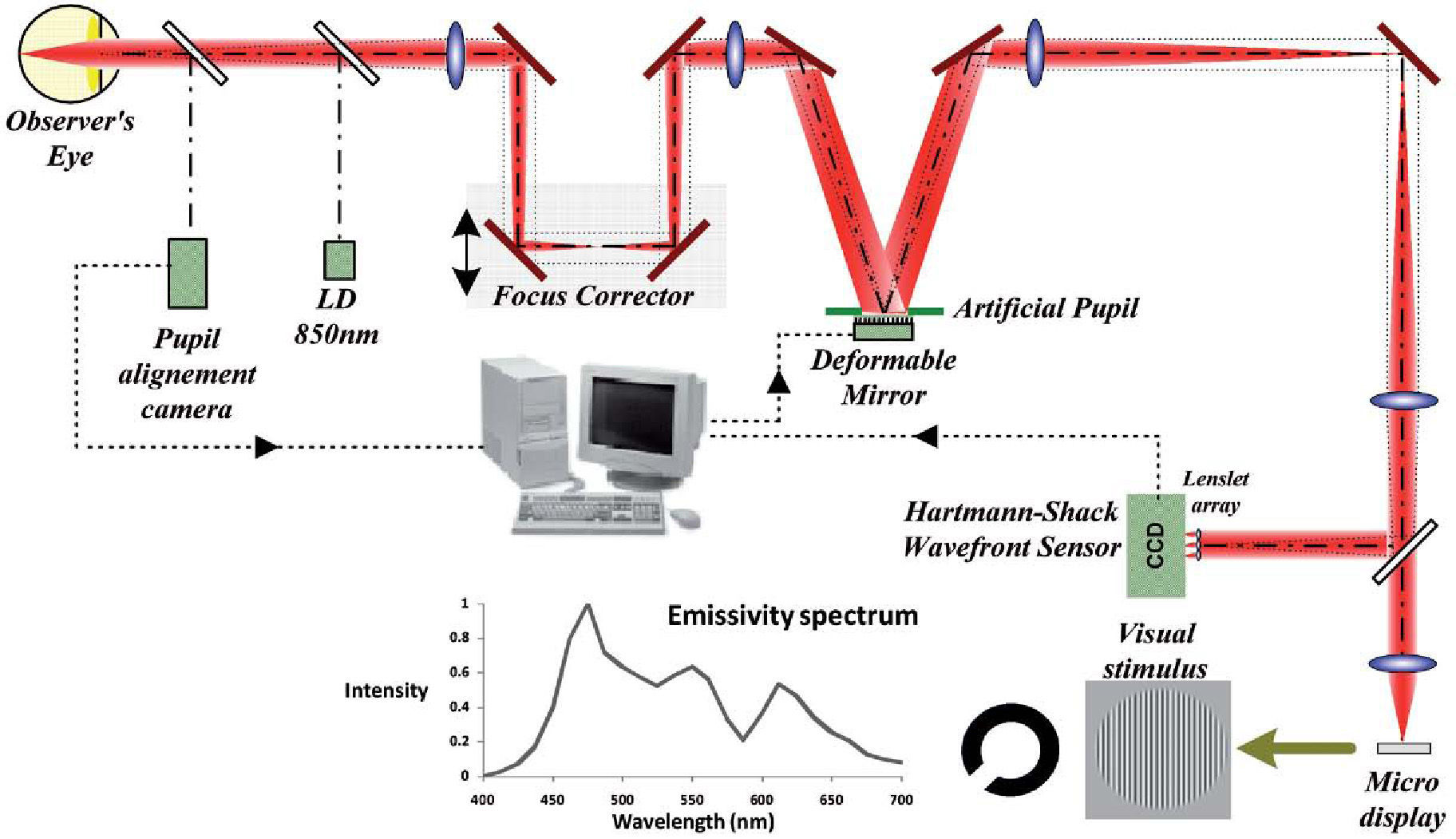
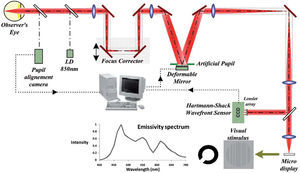
ApparatusWe used the CRX1© device (Imagine Eyes, Orsay, France) to measure and correct the wavefront aberration. This adaptive optics system is composed of two basic elements, which are the wavefront sensor and a correcting device. Figure 1 shows the schematic diagram of the AO device. The system optically conjugates the exit pupil plane of the subject with the correcting device, the wavefront sensor and an artificial pupil. The Shack-Hartmann wavefront sensor has a square array of 1024 lenslets. The wave-aberration measurements are made at 850nm. The wavefront correcting device is a deformable mirror having 52 independent magnetic actuators. The control of the deformable mirror surface is accomplished by a commercially available program (HASO© CSO, Imagine Eyes, Orsay, France) which reshapes the deformable mirror from its normally flat surface to a shape that corrects the aberrations up to the 5th order (18 Zernike coefficients)22. The subject viewed the micro display (subtending a visual angle of 114 x 86 arcmin) through the adaptive optics system and through a 5.5 mm artificial pupil. The display was linearized using a Topcon BM3 luminance meter, its mean luminance was 42 cd/m2, which corresponds to a retinal illuminance of 1000 Td at a 5.5 mm pupil diameter. The emissivity spectrum of the display was measured (see figure 1) and was taken into account in the metric calculations (see section on the calculation method). The pupil centre was aligned with the optical axis of the set-up, and its position was maintained using the control handwheel of the CRX1 device providing us a quick, smooth and fine adjustment. The pupil position and size was monitored using a CCD camera. The subject's pupil was not artificially dilated since the experiments were performed in dim surrounding illumination providing us with a subject's pupil diameter higher than 5.5mm and avoiding reflections.
Schematic diagram of the CRX1© system used to measure and correct the eye's aberrations. The device is composed of two basic elements: a Shack-Hartmann sensor and a correcting device (deformable mirror) conjugated to the pupil plane and to the two-dimensional lenslet array. Subjects viewed the micro-display through the deformable mirror and performed a contrast sensitivity or visual acuity test (picture in the lower right corner).
In a first step, three measurements of the wavefront aberrations were performed up to the 10th radial order (63 Zernike coefficients). Each astigmatism term (i.e. Z-22 and Z22) was averaged on the 3 measurements. In the 2nd-order-aberration-correction condition (i), this mean astigmatism was applied to the deformable mirror and maintained constant, independent of wavefront fluctuations that may occur in the eye under test. In both aberrations correction conditions, the subject used a Badal optometer (focus corrector) in order to adjust the defocus term and optimize the image quality of a 16c/deg vertical sinewave grating. We assumed that the defocus term was similar regardless of the high-contrast target used: sine-wave grating or Landolt-C. In the second aberration-correction condition (ii), aberrations up to the 5th order were dynamically compensated using a closed-loop system working at 3 Hz that comprises a double-pass of light through the eye, so that the total (eye-device) aberration encountered along the line of sight is continuously minimized. Consequently, condition (i) was actually a static correction of the 2nd order aberrations whereas in condition (ii), the 2nd order aberrations were dynamically corrected.
The level of the higher-order wave aberrations was 0.27±0.14μm RMS when averaged across the population. The typical residual astigmatism measured in the first aberration-correction condition (i) was less than 0.1 D, corresponding to 0.08μm RMS on a 5.5mm pupil. During the 5th order aberration-correction condition (ii), the average variance of the residual aberration was 0.09±0.02μm.
Measuring the Visual PerformanceThe 16 c/deg contrast sensitivities were measured using computer-generated randomly oriented sine-wave gratings. The subject's task was to identify the grating's orientation which could be horizontal, vertical, at 45° or at 135° (four-alternative forced-choice method) and to respond on a numeric keypad. To estimate the contrast threshold, a modified best Parameter Estimation by Sequential Testing (PEST) procedure was used. Measurements ended after 30 trials. The sinusoidal gratings were truncated by a windowing function which consists of a circular window subtending a visual angle of 1° surrounded by a sinusoidal function subtending a visual angle of 0.14° to smooth the edge of the field. The stimulus was presented for 500ms. The average of the 3 contrast threshold measurements was retained.
We used the Freiburg Acuity Test (FrACT) software23 to measure the subject's high-contrast visual acuity. The subject had to identify the position of a Landolt-C's gap; eight positions were possible (eight-alternative forced-choice method). To estimate the acuity threshold, a best PEST procedure was employed, the test ending after 30 optotype presentations. The average of 3 measurements was retained as the threshold acuity.
Calculating the One-dimensional MTFThe visual benefit calculations were based on metrics derived from the one-dimensional MTF in white light which was obtained by averaging the two-dimensional MTF across all orientations. The two-dimensional white light MTF was computed as the modulus of the Fourier transform of the polychromatic Point Spread Function (PSF). The polychromatic PSF was the sum of the individual monochromatic PSFs (PSF(λ)), weighted by V(λ), the photopic spectral luminous sensitivity of the eye as defined by the Commission Internationale de l’Eclairage (CIE) in 1924, and by E(λ), which is the emissivity spectrum of the display (see figure 1), i.e. Σλ[PSF(λ) × V(λ) × E(λ)]. The monochromatic PSFs were calculated for wavelengths from 400 to 700 nm in 20nm steps. In our method, the chromatic aberrations, which vary little between subjects24, always came from experimentally-based numerical models25,26, while the monochromatic aberrations, which show substantial inter-subject variations5,27 and are pupil-size dependent, came from wavefront aberration measurements of the eye measured in the three conditions described earlier (i.e. no correction, 2nd order correction and correction of the aberrations up to 5th order). The last part of the input data involved the detection parameters. Since we wish to simulate the photopic vision of individuals, we need to take into account some parameters concerning the detection of the image by the cones. The first stage of the detection process is included in our calculation and allows for the Stiles-Crawford effect, meaning that the rays coming from the more peripheral pupil area are less effective than the ones coming from the pupil centre. Mathematically, the Stiles-Crawford effect is introduced as an apodization of the pupil, by multiplying the wavefront function by the following factor (Applegate and Lakshminarayaman28):
where ρ is the attenuation factor (ρ=0.05mm−2), and xSCE, ySCE are the coordinates of the peak of transmittance. The peak of transmittance (xSCE, ySCE) is respectively decentred nasally and superior 0.4 and 0.2 mm.Predicting the Visual BenefitTo be compared to the measured contrast threshold visual benefit, we computed the sphero-cylindrical corrected MTF (MTFSC) and the 5th-order aberration-corrected MTF (MTFHO), the ratio between the 16 c/deg MTFHO and the 16 c/deg MTFSC was then calculated, i.e. MTF16c/degHO/MTF16c/degSC.
To be compared to the measured MAR visual benefit, various metric ratios were calculated including: (i) the ratio of the intersections between the MTF and a neural contrast threshold function measured by Williams29, i.e. intersectionHO /intersectionSC, (ii) the ratio between the volumes (between 0 and 60 c/deg) under the predicted CSF which is defined as the MTF weighted by a neural sensitivity function (CSFN), where CS = MTF * CSN computed at each spatial frequency, i.e. CSFvolumeHO/CSFvolumeSC, and (iii) the ratio of the volumes (between 0 and 60 c/deg) under the MTFs, i.e. MTFvolumeHO/MTFvolumeSC.
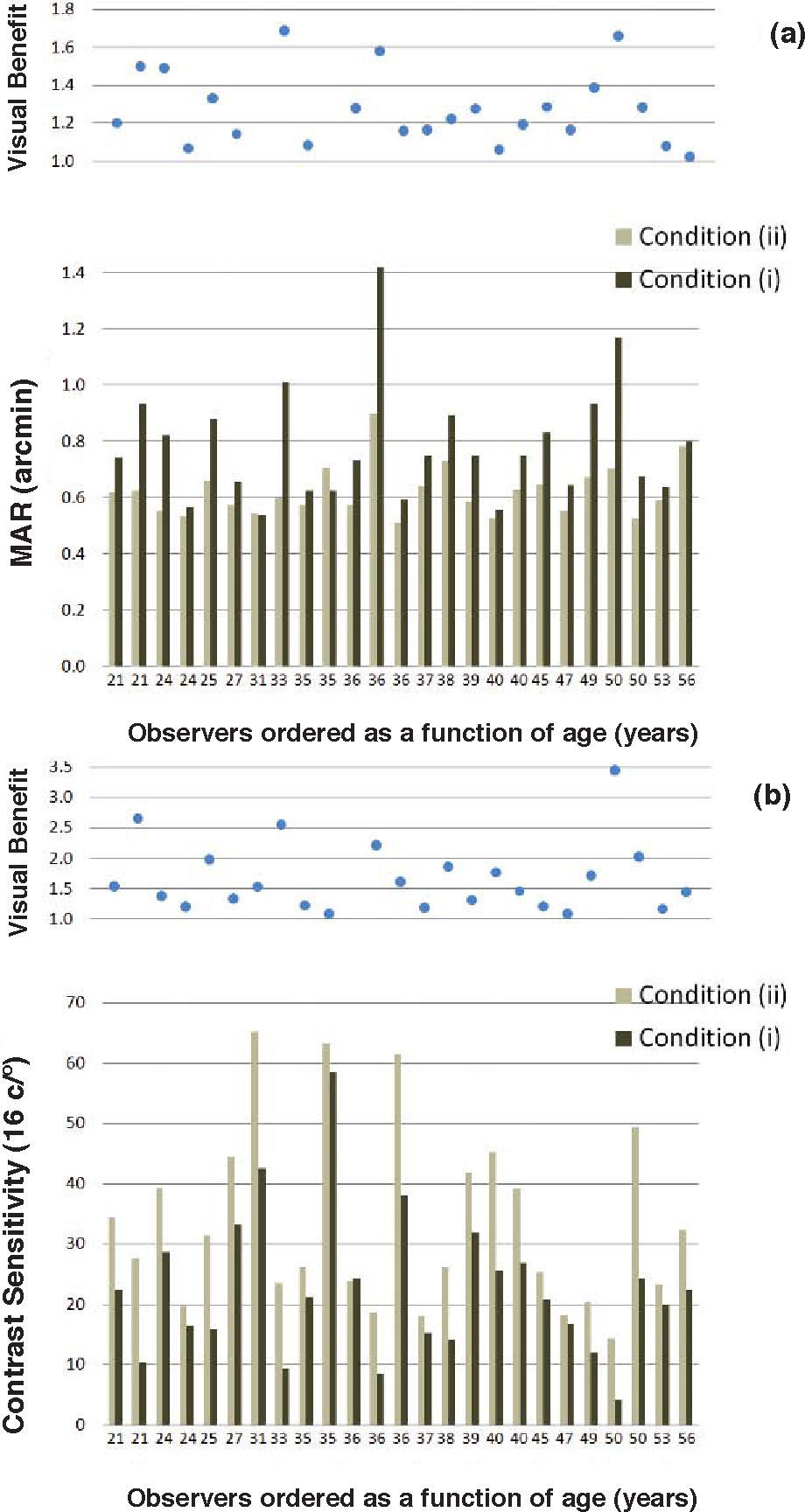
ResultsMean contrast sensitivity and minimum angle of resolution (MAR) measured in condition (i) were respectively 22.51 (SEM=2.29) and 0.78’ (SEM=0.04’) corresponding to a Snellen acuity of around 20/15.6. Mean contrast sensitivity and MAR measured in condition (ii) were respectively 33.26 (SEM = 2.95) and 0.62’ (SEM=0.02’) corresponding to a Snellen acuity of 20/12.4. Consequently, the averag visual benefits of correcting the aberrations up to the 5th order were 1.64 (SEM=0.12) and 1.25 (SEM=0.04) respectively in terms of contrast sensitivity and visual acuity. CS and MAR measured in both conditions of correction are represented in figure 2 for all the observers ordered as a function of their age, and the corresponding VB is also shown.
Measured MAR (a) and contrast sensitivity (b) for all the observers ordered as a function of their age with (grey bars) and without (black bars) AO correction. The upper part of the graph shows the corresponding measured visual benefit defined as the ratio of the visual performances measured in condition (i) and (ii). A ratio higher than 1 means that the performance is improved when correcting all the monochromatic aberrations up to the 5th order (i.e. condition (ii)).
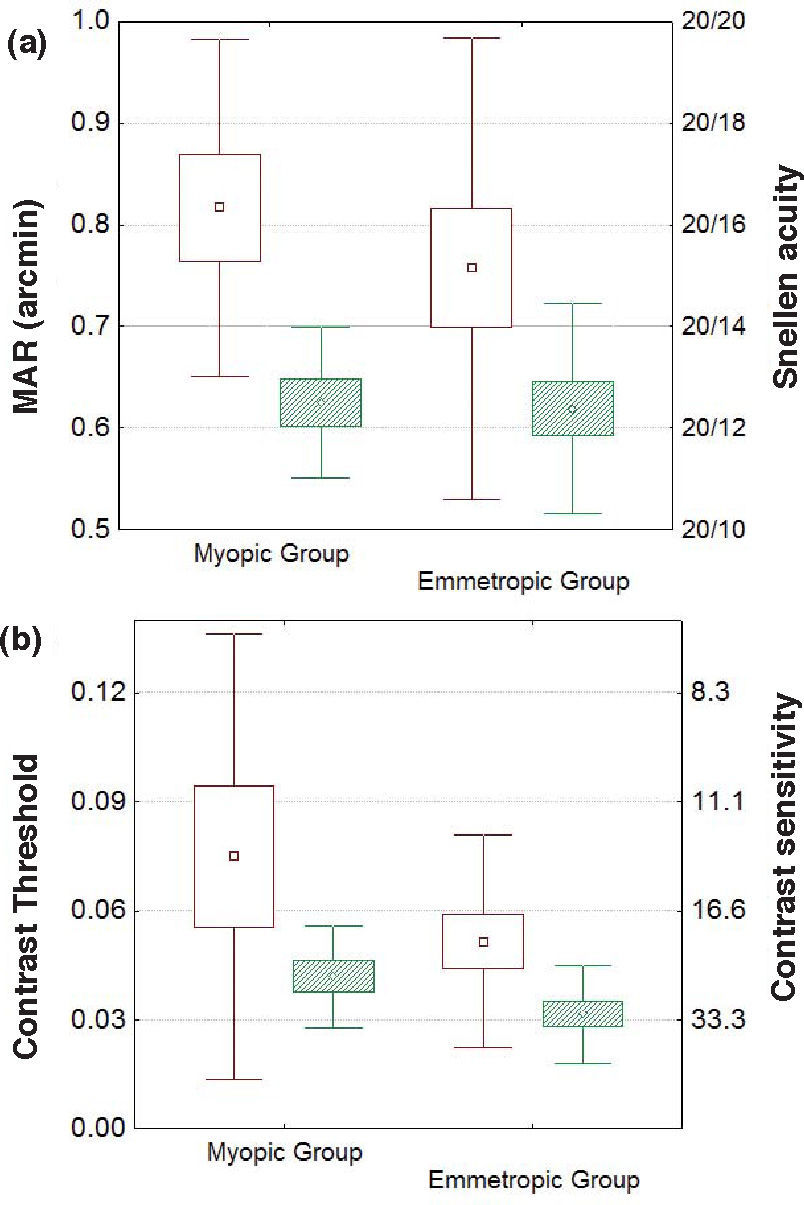
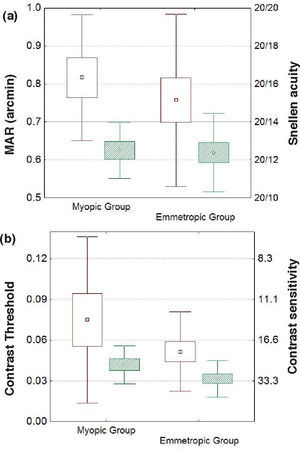
CS and MAR measured in conditions (i) and (ii) are shown in figure 3 for the myopic group (n=10) and for the emmetropic group (n=15). Mean contrast sensitivity VB was respectively 1.68 (SEM=0.25) and 1.61 (SEM=0.11) for the myopes and emmetropes. The difference between groups was not significant (P=0.056, t test). In term of visual acuity, the average VB was 1.31 (SEM=0.07) for the myopic group and 1.21 (SEM=0.05) for the emmetropic group. The difference between groups was not significant (P=0.442, t test). There was no difference (P=0.11, t test) in the MAR, measured in condition (ii), between emmetropes and myopes. It should be noticed that the variability of visual performances across individuals was at least twice as large when measured in condition (i). This result can be explained by the variability of the level of HOA across subjects, which was respectively 0.14 and 0.02μm in condition (i) and (ii).
Averaged measured MAR (a) and contrast sensitivity (b) for the myopic group and the emmetropic group in condition (ii) (dashed green squares) and in condition (i) (open red squares). The box and the error bars represent respectively the standard error and the standard deviation of the mean.
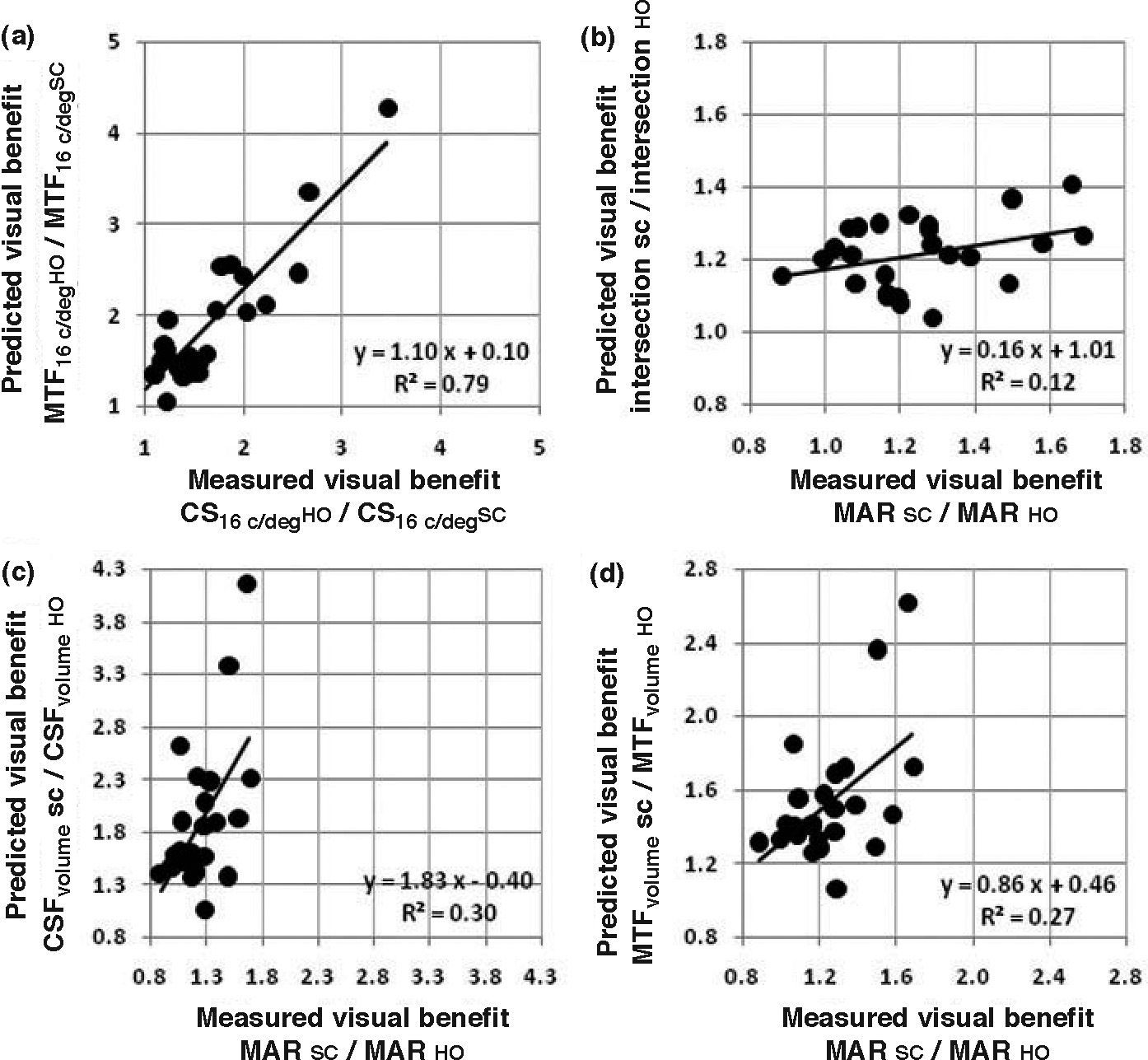
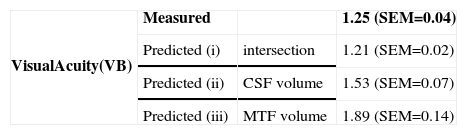
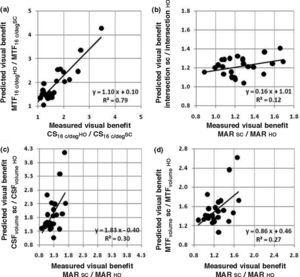
Figure 4 shows the correlation for the 25 subjects between the measured visual benefit and the value predicted by different metrics: (a) concerning the contrast sensitivity and (b), (c) and (d) concerning the visual acuity. Measured 16 c/deg-contrast sensitivity visual benefits were well correlated (r2=0.79) with VBs defined as the ratio of the modulation transfer function calculated at 16 c/deg in both conditions of aberration correction. None of the three calculated VB was found to be well correlated to the measured MAR improvement. The highest correlation (r2=0.30) was obtained with the metric where the MTF is weighted by the neural contrast sensitivity function (CSFN). However, the average VB on the population predicted by the intersection between the MTF and the neural threshold curve was comparable to the measured VB (see table 1).
We measured a 1.25-fold and a 1.64-fold improvement respectively of the visual acuity of the contrast sensitivity measured at 16 c/deg when measuring the visual performances in condition (ii): dynamic correction of the aberrations up to the 5th order in polychromatic light with a 5.5 mm pupil size. Since the 2nd order aberration were statically corrected in condition (i) and dynamically corrected in condition (ii), this may explain a small part of the visual benefit measured in condition (ii).
As in the Guirao et al.’s calculations5, we measured important variations of VB across eyes with some eyes showing no benefit whereas others obtained a contrast sensitivity benefit higher than 3 or a visual acuity benefit higher than 1.6. Our measured VBs were comparable to the 1.9-fold and 1.2-fold improvement respectively in CS and VA measured by Yoon and Williams3 respectively on 2 and 7 subjects with a 6 mm pupil size. Poonja et al.4 and Rossi et al.6 obtained a visual acuity VB in monochromatic light of around 1.5. The difference of VB between these studies may be explained by the absence or presence of chromatic aberration. In fact, it has been suggested already that the impact of the correction of monochromatic aberrations could be enhanced in the absence of chromatic aberration. In fact, Yoon and Williams, MTF calculations3, based on the measured wave aberrations of 17 normal subjects, showed that the VB of correcting the monochromatic aberrations was twice as large as when computed in absence of chromatic aberration.
We did not find any correlation between the VB and age (see figure 2), between age and the high-order aberration magnitude (r2=0.04), between age and MTF-based metrics (r2<0.1) and nor between age and the visual performances measured in condition (ii) (i.e. r2=0.04 with MAR and r2=0.03 with CS). This last result indicates that the post-receptoral performance did not vary with age and corroborates the results of Dressler and Rassow30 and Kayazawa et al.31 who did not find any significant influence of the age on the neural contrast sensitivity function. While Rossi et al.6 found a significant lower visual benefit in the myopic group, we did not find any statistical difference. The main difference between Rossi's results and ours concerns the MAR measured in condition (ii) in the emmetropic group, since we found an average MAR of 0.62’ instead of 0.49’ for Rossi. Visual stimulus delivery in AOSLO allows the presentation of stimuli of higher optical quality, probably better than ours since we did not avoid the degradation of the retinal image induced by chromatic aberrations, which prevented us from obtaining a visual acuity closer to the limit of resolution of the retina derived from the photoreceptor spacing at the fovea (i.e. Mean= 0.51’, a Snellen acuity of 20/10.2, Yoon and Williams3).
The ratio of the 16 c/deg MTF computed in the two conditions of aberrations correction (i.e. MTFHO / MTFSC) is well correlated with the measured contrast sensitivity VB meaning that this metric is able to predict the effects of aberrations on contrast sensitivity. To our knowledge, only Yoon and Williams3 both measured and calculated the VB in term of contrast sensitivity. However, on the 2 subjects involved in their experiment, measurements and predictions were quite different. Since the contrast sensitivity can be well predicted by a metric based on wave aberration measurements, the neural adaptation to monochromatic aberrations first proposed by Artal7 does not seem to have played such an important role in our experiment. The hypothesis of a cortical adaptation to blur has been suggested to explain subjective preference to one's own aberration. However, this hypothetic effect has never been observed in term of contrast sensitivity measurements. In conclusion, this question requires further investigation.
We did not obtain the same quality of prediction with visual acuity. The best correlations were r2=0.30 and r2=0.27 respectively when computing the ratio of the volumes under the predicted CSFs (i.e. CSFHO / CSFSC) and when calculating the ratio of the intersections between the MTF and the CSFN. Both metrics involved the neural contrast sensitivity function measured by Williams29 on six subjects. Since the CSFN shows large inter-individual variation30-32 the inability to predict visual performances of individuals using data averaged on a population is not surprising. However, the intersection metric predicted an averaged VB of 1.21 (SEM=0.04) compared to the averaged measured VB of 1.25 (SEM = 0.02). This good agreement confirms that general tendencies of a population could be predicted by metrics taking into account a “standard” CSFN, whereas predictions for an individual requires a measurement of its own CSFN. Then, in this case it is better to directly measure the visual performance instead of predicting it via another measurement. An intermediate solution could be the use of typical CSFN of a given population. The typical CSFN should be categorized as a function of parameters such as retinal illuminance, age or ametropia. Consequently, the use of such categorized neural contrast sensitivity functions should improve the accuracy of the prediction of the visual acuity. However, part of the reason behind the better predictability of the CS could be explained by the inherent differences between the demands of the VA and CS tasks. Indeed, the VA task involves a more complex neural process than a task where the subject only needs to detect and recognize the direction of a grid (i.e. CS measurement).
In conclusion, metrics based on wave aberration measurements are able to predict the impact of monochromatic aberrations on contrast sensitivity and should be able to predict its effect on visual acuity with the use of categorized neural contrast sensitivity functions. This simulation should be considered as a useful tool for optical designers since it allows to predict the effect of optics (i.e. impact of aberrations) on visual performances without the need to manufacture prototypes, hence saving time and money.