To enhance the accuracy of intraocular lens (IOL) power calculation in patients with Fuchs’ endothelial corneal dystrophy (FECD) undergoing simultaneous cataract surgery and Descemet membrane endothelial keratoplasty (triple-DMEK) by predicting corneal power changes.
MethodsObservational ambispective monocentric cohort study. A linear corneal change model (LCCM) was developed to predict corneal change from the preoperative corneal ratio (anterior/posterior radius). LCCM was validated by comparing prediction errors with the traditional IOL optimization method.
Results97 eyes of 69 patients were analyzed. Preoperative keratometry was biometrically unmeasurable in 9 eyes, so manually entered autorefractometer data were used for IOL calculations and were analyzed separately. Mean absolute error (MAE) in the manual group (1.35 D (-1.04, 3.75)) was higher than the measured group (0.75 D (-0.62, 2.12)). The median change in simulated keratometry (SimK) was -0.21 ± 0.68 D and in total keratometry (TK) was -0.62 ± 1.09 D (p < 0.001). SRKT outperformed the rest with constant optimization (0.60 D (-0.53, 1.74)). LCCM showed similar MAE to the constant optimization method (p > 0.05). However, MAE for the optimization method was higher (2.08 D (1.77, 2.39)) than LCCM method (1.87 D (1.62, 2.12)).
ConclusionsSimK and TK change significantly after Triple-DMEK. The LCCM could reduce extreme refractive surprises by assisting surgeons in the individualized selection of the best IOL for each eye based on the expected corneal change. Study limitations include variability in FECD severity and the inherent limitations of biometric formulas applied to non-standard eyes. Further studies are recommended.
Since the inception of intraocular lens (IOL) implantation in cataract surgery, starting with the pioneering case performed by Sir Harold Ridley in 1949, the need for a method to calculate the proper power of the IOL to be implanted in order to minimize the resultant refractive error arose.1 In subsequent years, different IOL calculation formulas came up: historical/refraction based, that are obsolete; theoretical, based on the application of laws of Gaussian geometrical optics to an optic model (also schematic eye); empirical, based on the retrospective study of the refractive result of multiple surgical interventions and performing regression statistical analysis; and more modern formulas based on artificial intelligence and ray tracing.2 It was Fyodorov in 1967 who described the first theoretical IOL calculation formula.3 Since then, formulas have significantly evolved, leading to improved refractive outcomes in terms of postoperative spherical equivalent (SE) achieved. Currently, the traditional and newer-generation theoretical formulas are considered the most accurate, as they exhibit fewer predictive errors compared to empirical ones, particularly in eyes with extreme axial length (AL) and mean corneal power (Km) values.4
IOL power assessment is based on multiple variables, including preoperative ocular measurements which are unique and individual to each patient, being Km and AL the principal parameters, along with specific IOL model characteristics.2,5 The effective lens position (ELP), defined as the postoperative distance between the corneal apex and the main plane of the IOL (assumed without thickness), is the only measurement that cannot be determined preoperatively. This poses a significant challenge for the various theoretical vergence formulas, as it has been identified as the primary source of error in IOL calculation.6 Researchers have developed new formulas with enhanced algorithms to address ELP prediction, increasingly incorporating a broader range of parameters, primarly biometric measurements. Moreover, they have incorporated an adjustment factor known as the lens constant into their formulation. The value of the constants is different for each IOL model depending on its optical properties, considering its specific optical geometry and the angulation of its haptics. The use of optimized lens constants have been proven to enhance refractive outcomes.7
On the other hand, postoperative decrease of the total keratometry (TK) following Descemet membrane endothelial keratoplasty (DMEK) has been linked to an increase in posterior corneal surface curvature as a consequence of reversal of corneal edema, while the anterior corneal surface appears to have no significant influence on this phenomenon.8,9 This aspect should be considered in patients who are candidates for cataract surgery either before or simultaneously with DMEK (Triple-DMEK) to prevent hyperopic surprises.8–10
The aim of this study is to refine the accuracy of IOL power calculation by optimizing the lens constants of different hydrophobic IOL models in patients undergoing triple-DMEK, in order to improve their refractive outcomes. As a secondary objective, we will assess the potential impact of predicting the corneal power change induced by triple-DMEK procedure and taking it into account for IOL calculation with the diverse formulas, in comparison with the accuracy of the previous more traditional pre-fitting method by analyzing their prediction errors. All this with the ultimate goal that the choice of the IOL power is more objective being based on an adjusted calculated target and not on a subjective election by the surgeon of a proposed range to avoid refractive surprises.
MethodsWe conducted an observational ambispective monocentric cohort study of all patients with Fuchs’ endothelial corneal dystrophy (FECD) that underwent uneventful triple-DMEK at Fundación Jiménez Díaz University Hospital, a Spanish tertiary care referral centre, from the last 5 years (2018–2022), with a minimum follow-up of 3 months.
EthicsThis study followed the tenets of the Declaration of Helsinki and the study protocol was approved by the Institutional Review Board and Ethics Committee of the Fundación Jiménez Díaz.
Exclusion criteriaPatients with causes of endothelial dysfunction other than FECD (such as herpetic endothelitis or iridocorneal endothelial syndrome) or with history of corneal injuries, ocular infection, ocular inflammation or refractive surgery were excluded. Cases with preoperative ultrasound biometry or with low visual acuity that made difficult performing postoperative subjective refraction were also excluded.
Data aggregationSome cases in the sample of this study contain incomplete or missing data. To clarify and prevent errors in the analysis, different study groups were created for hypothesis testing. For instance, when comparing errors in the biometric formulas, patients with manually entered preoperative keratometry data (manual group) were separated from those with keratometric biometric inputs. Similarly, when analyzing corneal changes, only the cases with available anterior corneal surface information (pre- and postoperative Simk) were used for SimK change calculation. On the other hand, only those patients with available pre- and postoperative information of both corneal surfaces (pre- and postoperative SimK and corneal ratio) were included for TK change analysis. Finally, to develop the fitting model based on corneal change, hereafter referred to as the linear corneal change model (LCCM), only the cases with pre- and postoperative measurements with information on both corneal surfaces and labelled as “Successful” by the biometer were used. In addition, for the comparison between the optimization method and the LCCM, only data from those patients with Bi-Flex (Medicontur) IOL and available preoperative corneal ratio were used.
VariablesThe collected data from patient's medical records included the following: demographic information (age and sex), best corrected visual acuity (BCVA) in the latest clinical review prior to triple-DMEK, surgery date, specific hydrophobic monofocal IOL model implanted and clinical data obtained at 3 months or later after the surgery (including date, autorefractor measurements, subjective refraction and BCVA).
The remaining variables used in this study were obtained from the IOL Master 700 swept-source optical biometer (Carl Zeiss Meditec AG, Jena, Germany). Pre- and postoperative measurements were collected, for a minimum of 3 months after the surgery in the latter case. These measurements included AL, anterior chamber depth (ACD) and the principal meridians of both the anterior and posterior corneal surfaces (flat K and steep K). For convenience and clarity, the mean radius of the anterior and posterior corneal surfaces were converted from millimetres to power expressed in diopters (D). The front corneal power determined through simulated keratometry (SimK), was derived from the front radius and the standard keratometric refractive index of 1.3375. The power of the whole cornea, encompassing both the anterior and posterior surfaces, was assessed through TK measurements. To describe those variables, the median and interquartile range was used due to skew distribution.
The corneal ratio was calculated as the ratio between the front and back corneal radius in both the pre- and postoperative scenarios. The change in this variable was determined by subtracting the preoperative ratio from the postoperative ratio.
Surgical techniqueTriple-DMEKs were performed by 3 experienced corneal surgeons (NAA, BGS and IJA) following the same standardized surgical technique that we have previously specified in a previously published article.11 One or the other IOL was chosen according to the refractive target (between −0.50D and −1.00D) and the surgeon's preference, as several models of hydrophobic IOLs were available.
Intraocular lens calculationsThe variables used for IOL calculations included the AL, the ACD and the mean SimK, obtained from the IOLMaster 700 (1.90.33.04 version). Classical formulas algorithms (SRK/T, Hoffer Q, Holladay I and Haigis) were programmed using routine algorithms in Python language and cross-checked with those given by IOL Master 700.12–15 New-generation formulas results were computed using on-line free calculators at date April 2023: Barrett Universal II formula V1.05 (https://calc.apacrs.org/barrett_universal2105/), Emmetropia Verifying Optical (EVO) IOL calculator V2.0 (https://www.evoiolcalculator.com/calculator.aspx), Kane formula V1.42 (https://www.iolformula.com/) and PEARL-DGS formula for regular eyes (https://iolsolver.com/regular). The initial constants for each formula were obtained from the IOLCon optimized constants database website (https://iolcon.org/). For the new formulas the optimization procedure was computed using A constant. The selection of these specific formulas and sources was based on their widespread acceptance and validation in the ophthalmology community, as well as their availability for free and easy access, ensuring comprehensive and up-to-date results for IOL calculations.
Assessment of formula errors and optimization procedureThe prediction error of each formula was analyzed by subtracting the predicted refraction (biometric target) from the residual subjective refraction, measured 3 months or more after Triple-DMEK, using SE notation. In addition, we also evaluated the mean error (ME) and mean absolute error (MAE), as suggested by best practice guidelines.16 The ME provides insight into the average difference between the predicted refraction by each formula and the postoperative residual subjective refraction, considering both positive and negative deviations. On the other hand, the MAE calculates the average absolute difference between the predicted and observed refraction, disregarding the direction of the deviation. Both measures are widely used in the literature to describe the formulas performance and reliability in IOL calculations. MAE values are expressed as mean (95% confidence interval).
Another commonly used method for evaluating the error, that we have also carried out, is to assess the number or percentage of eyes with a prediction error within various error intervals in D and visually represent the results using histograms. This approach provides a clear depiction of the distribution and frequency of errors across the sample, allowing for a comprehensive understanding of the accuracy of the formulas.
The constant optimization was assessed adding the ME to the initial constant (obtained from IOLCon). Then, this new prospective constant was used to obtain a new ME. This iterative procedure was repeated until the ME reached a threshold of <0.01D At this point, we determined that the constant had been optimized. The optimized constants were only assessed for the Bi-Flex HB-877FAB (Medicontur Medical Engineering Ltd., Inc., Zsambek, Hungary) and AcrySof-IQ SN60WF (Alcon Laboratories, Inc., Fort Worth, TX, USA) IOL models using routine algorithms.17
Corneal refractive changes induced by triple-DMEKFirstly, we conducted a univariate analysis using the Wilcoxon signed-ranked test to investigate the changes in SimK and TK separately. A p-value < 0.05 was considered statistically significant.
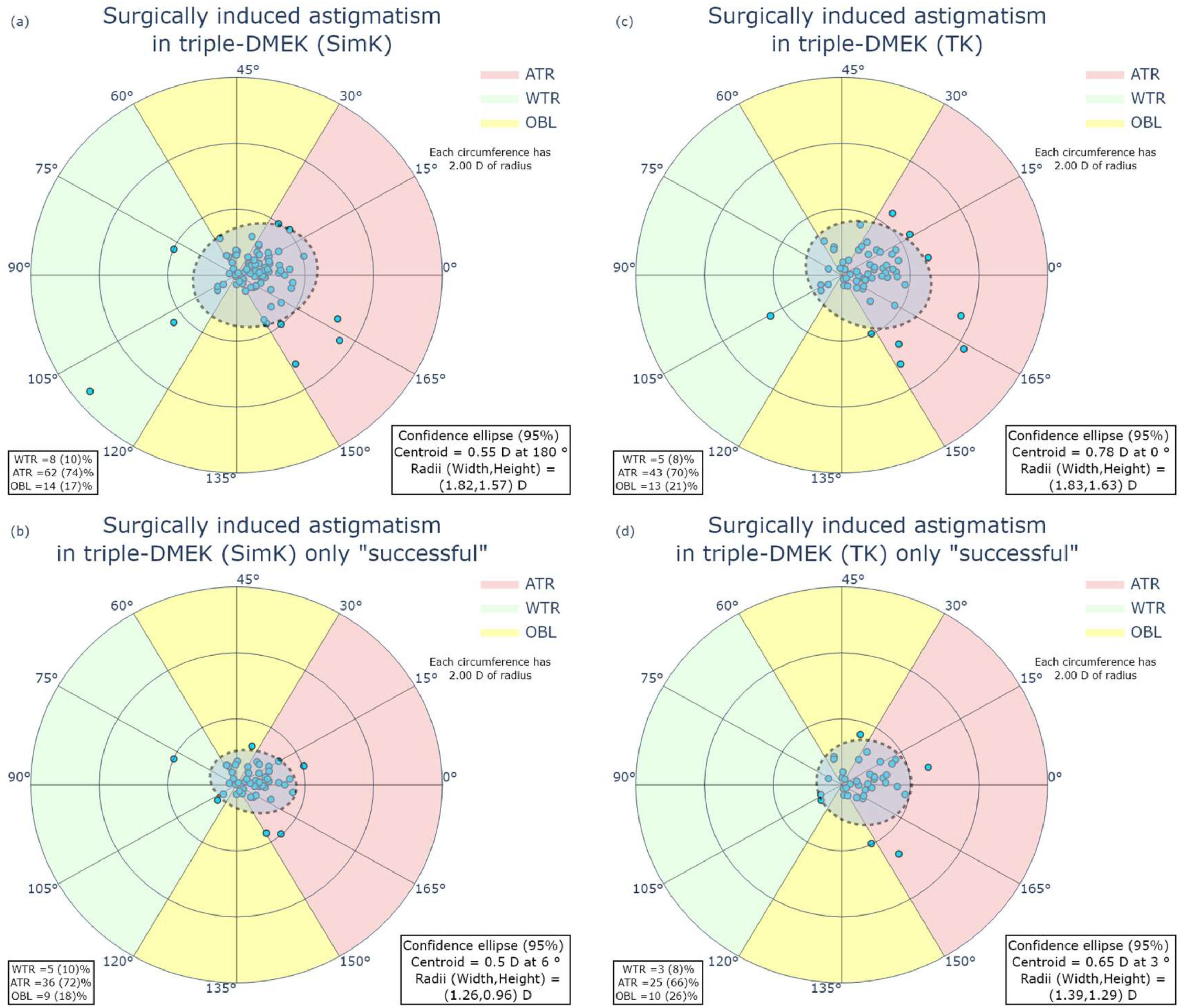
Astigmatism was analysed using the power vectors method (J0 and J45 vectors). J0 represents the horizontal cylindrical component of astigmatism and, if it is positive, it means that the astigmatism is with-the-rule (WTR), while if it is negative is against-the-rule (ATR). J45 represents the oblique cylindrical component of astigmatism, a positive value indicates oblique astigmatism with its principal axis at 45°, while a negative value indicates principal axis at 135°. For the analysis of the results, we used statistical description of those mentioned vectors on the pre- and postoperative stage. Also, the astigmatic change was computed as the subtraction (postoperative - preoperative) and it was visually represented on the double angle vector diagrams (DAVD) using the positive cylinder notation with the 95% confidence ellipse of the data.18,19 The same analysis were performed for a filtered database which included only those measurements labelled as “Successful” by the IOLMaster 700 device.
Modelling corneal power changeThe modelling process was obtained utilizing the filtered database that exclusively included "Successful" biometric measurements of eyes with pre- and postoperative data of both corneal surfaces, ensuring the validity of the data. To analyse the correlation between variables that describe the corneal change (TK and SimK) and the preoperative variables (preoperative corneal ratio (CRpreop)), a least square linear model was tested. The robustness of the model was evaluated using the coefficient of determination (R2), with a result > 0.4 considered a moderate correlation. By means of this, we aimed to gain insights into the underlying factors affecting corneal changes. The formulas to obtain the corneal change prediction are depicted on Fig. 1. The difference in correlation between the middle graph for TK (R² = 0.39) and the right graph for SimK (R² = 0.28) suggests that the posterior corneal surface plays a significant role in corneal change. For this reason, the formula chosen to estimate corneal change was: estimated TK change = +12.88 × CRpreop - 14.74. A negative value will indicate that the cornea loses power after the surgery, and this value is the one that will indicate the target we should aim for with the selected biometric formula. To see an example that helps to understand its application, consult the Supplementary material.
Linear least squares of the preoperative variables that showed strongest correlation with the corneal change.aa Total Keratometry (TK), Simulated Keratometry (SimK).
To compute the change in corneal power the pre- and postoperative corneal ratio are needed (real TK change = CRpostop - CRpreop). The corneal ratio is obtained by dividing the front corneal radius by the back corneal radius, and it provides information about the relative curvatures of the anterior and posterior corneal surfaces. In the literature, the normal value for the corneal ratio has been commonly defined around 1.2.6,20 Changes in the corneal ratio can indicate alterations in one or both corneal curvatures, thus considering only the SimK to determine the corneal refractive power might be an important source of error in patients with an altered corneal ratio.
To assess the LCCM error for each formula, the corneal change prediction (estimated TK change) is needed. As mentioned above, this value is obtained by a linear regression model using the CRpreop and the formula that is located in the upper left corner of the middle graphs in Fig. 1. Finally, this change is subtracted from the formula expected refraction with the initial constant (not optimized), and the result of this difference is subtracted from the real postoperative subjective refraction in SE notation. Thus, the error for the IOL calculation considering the corneal change can be assessed for the different formulas, as presented in the example showed in Supplementary material.
To evaluate the accuracy of the LCCM, which considers the corneal change in IOL calculation with the IOL constants from IOLCon, a comparative analysis was performed in eyes implanted with the Bi-Flex IOLs that exhibited corneal ratio data available. This involved comparing the LCCM results with those obtained by constant optimization for each formula. Descriptive statistical analysis was applied to evaluate absolute errors and error intervals.
ResultsSample descriptionDemographical and clinical data from the sample, including data availability, implanted hydrophobic IOL model and preoperative ocular biometry measurements are depicted in Table 1.
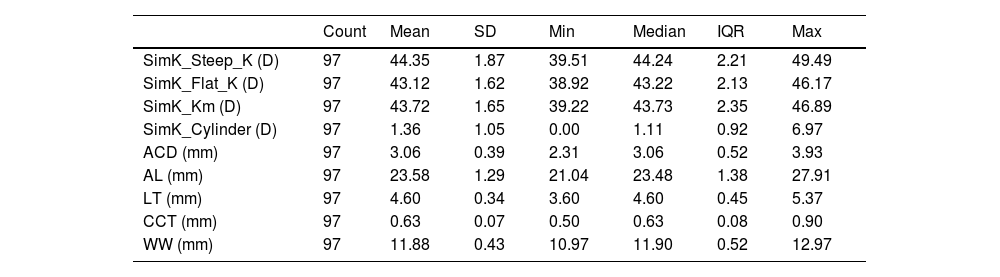
Descriptive data of biometric parameters for intraocular lens calculation.dd Simulated Keratometry (SimK), Diopters (D), Mean corneal power (Km), Anterior Chamber Depth (ACD), Millimeters (mm), Axial Length (AL), Lens Thickness (LT), Central Corneal Thickness (CCT), White-to-White corneal diameter (WW), Standard Deviation (SD), Minimum value (Min), Interquartile Range (IQR), Maximum value (Max).
When comparing the group of patients in which biometry inputs were available (measured group, N = 88) with the group of patients in which keratometric values from the autorefractometer were manually entered into the biometer due to measurement failures (manual group, N = 9), no notable differences were observed between them in the preoperative values. Also, the residual refraction in SE notation for both groups was similar, −0.05 ± 0.84 D for those eyes measured and −0.35 ± 1.81 D for the manual group. Selecting the Bi-Flex and SN60WF IOL models, the mean target refraction across all the formulas was −0.61 ± 0.3 D for the measured group (N = 63) and −0.95 ± 0.6 D for the manual group (N = 9). A notable MAE difference was found between the measured group and the manual group considering the average of the values obtained with the initial constants for the different formulas, 0.75 D (−0.62, 2.12) versus 1.35 D (−1.04, 3.75) respectively. No statistical tests were performed for these comparisons between both groups due to the difference in sample sizes. The value improves even further when analysing only the eyes in the measured group labeled as successful by the biometer: 0.69 D (−0.07, 1.45).
Corneal refractive changes induced by triple-dmekFor the analysis of the first corneal surface, we obtained the pre- and postoperative SimK from 84 eyes, as 4 eyes of the measured group (N = 88) had no postoperative information. In this group, the SimK change was −0.21 ± 0.68 D Similarly, considering only those eyes where the quality of the keratometry measurement was “Successful” (N = 50), the change was −0,21 ± 0.51 D In both cases the SimK change was statistically significant (p < 0.001). On the other hand, the manual group (N = 9) showed a SimK change of −0.3 ± 0.82 D, not reaching statistical significance (p = 0.31). Astigmatic power vectors J0 and J45, changed from 0.17 ± 0.68 D and 0.02 ± 0.45 D to −0.15 ± 0.65 D and −0.11 ± 0.48 D, respectively. More information is shown in Fig. 2a and b.
Double angle plotted diagrams showing front corneal astigmatism (2.a, N = 84) and total corneal astigmatism (2.c, N = 61) induced by Triple-DMEK considering all eyes with available postsurgical data and front corneal astigmatism (2.b, N = 50) and total corneal astigmatism (2.d, N = 38) considering only "Successful" measurements determined by the IOLMaster 700 biometer.bb Simulated Keratometry (SimK), Total Keratometry (TK), Against-The-Rule astigmatism (ATR), With-The-Rule astigmatism (WTR), Oblique astigmatism (OBL), Diopters (D).
The pre- and postoperative data for the whole cornea were available for 61 eyes. In this group, the TK change was −0.62 ± 1.09 D For the subgroup with optimal keratometry readings (N = 38), this change was −0.63 ± 0.78 D In both cases the change was also statistically significant (p < 0.001). Consistent with the astigmatism in SimK, the preoperative TK power vectors J0 and J45 were 0.18 ± 0.58 D and −0.07 ± 0.53 D, while postoperatively changed to −0.23 ± 0.67 D and −0.12 ± 0.49 D More information is available in Fig. 2c and d.
In both SimK and TK DAVDs (Fig. 2), a trend toward against the rule (ATR) astigmatism induction was observed. Moreover, a reduction of around 0.5 D in the magnitude of both vertical and horizontal axis of the confidence ellipse when considering only the successfully measured eyes, suggests a more accurate value of the surgical induced astigmatism (SIA). To evaluate the changes in the SimK and TK cylinders we conducted a univariate and multivariate analysis. In the univariate analysis, when comparing the SimK cylinder change, the preoperative and postoperative J0 vector showed statistically significant differences using the paired-sample Student's t-test (p < 0.001, T = 5.39), while the oblique component J45 did not (p = 0.97, T=−0.04). Similarly, the TK cylinder J0 component changed significantly (p < 0.001, T = 6.54), whereas J45 did not (p = 0.92, T = 0.09). The multivariate analysis (preoperative J0 and J45 versus their postoperative counterparts) confirmed the previous findings. The Hotelling's T-squared test showed statistically significant differences for both SimK (p < 0.001, T²=40.27) and TK (p < 0.001, T²=44.41). These results suggest that Triple-DMEK could significantly induce ATR astigmatism.
To assess a regression model that could predict the corneal power change, only those eyes with optimal quality measurements from both corneal surfaces were selected (N = 38). The preoperative feature with the highest determination coefficient was the corneal ratio. There was an inverse weak correlation between the corneal ratio and the SimK change (R2 = 0.28). This correlation increases when considering the TK change (R2 = 0.39). Also, the change in corneal ratio and preoperative corneal ratio showed a moderate correlation (R2 = 0.42). The equations are depicted in Fig. 1.
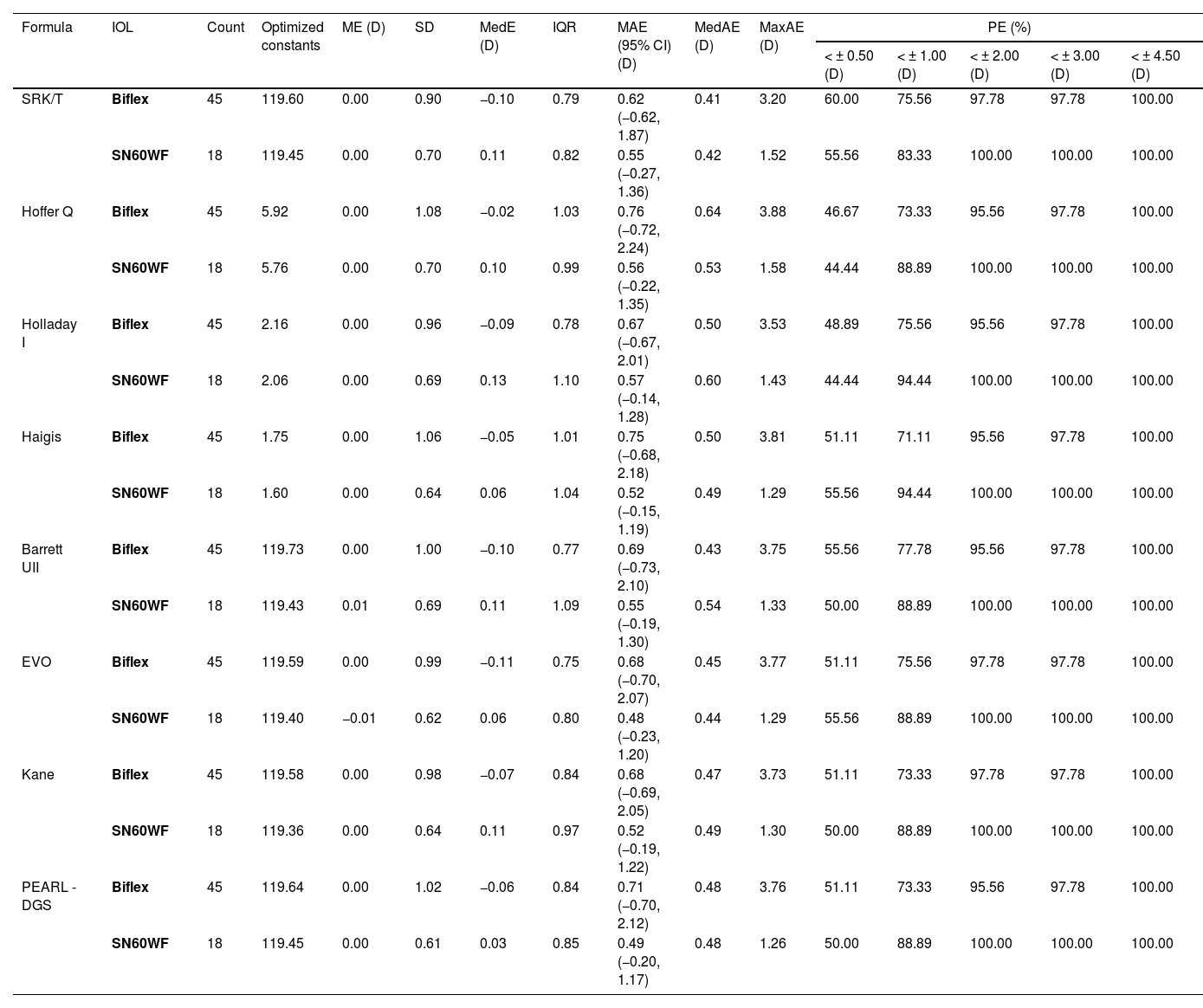
Assessment of formula errors and optimization procedureFirst, we analysed the results obtained with the initial (non-optimized) constants and the selection of the Bi-Flex (N = 45) or SN60WF (N = 18) IOL power for a target of −0.61 ± 0.30 D The MAE was similar across all formulas (0.75 D (−0.62, 2.12)). Before constant optimization, the formula with the lowest MAE was EVO (0.69 D (−0.69, 2.06)) while Haigis and Holladay I obtained the highest MAE values (0.80 D (−0.62, 2.22) and 0.80 D (−0.55, 2.16), respectively) (p < 0.001). For a thorough analysis segregated by IOL model, please consult the table 2. The manual group showed higher values of MAE than the measured group, being the SRK/T formula the one that exhibited the lowest value (1.23 D (−1.13, 3.60) and the Haigis the one that displayed the highest value (1.50 D (−0.78, 3.77)). In contrast, the eyes in the measured group labeled as successful by the biometer showed lower MAE values, with the Kane formula yielding the best value (0.60 D (−0.13, 1.34)) and the Holladay I formula displaying the highest value (0.81 D (0.08, 1.54)).
Prediction error analysis for Biflex and SN60WF lens models for different formulae using initial constants from IOLCon.ee Barrett Universal II (Barrett UII), Emmetropia Verifying Optical (EVO), Intraocular Lens (IOL), Mean Error (ME), Diopters (D), Standard Deviation (SD), Median Error (MedE), Interquartile Range (IQR), Mean Absolute Error (MAE), 95% Confidence Interval (95% CI), Median Absolute Error (MedAE), Maximum Absolute Error (MaxAE), Prediction Error (PE).
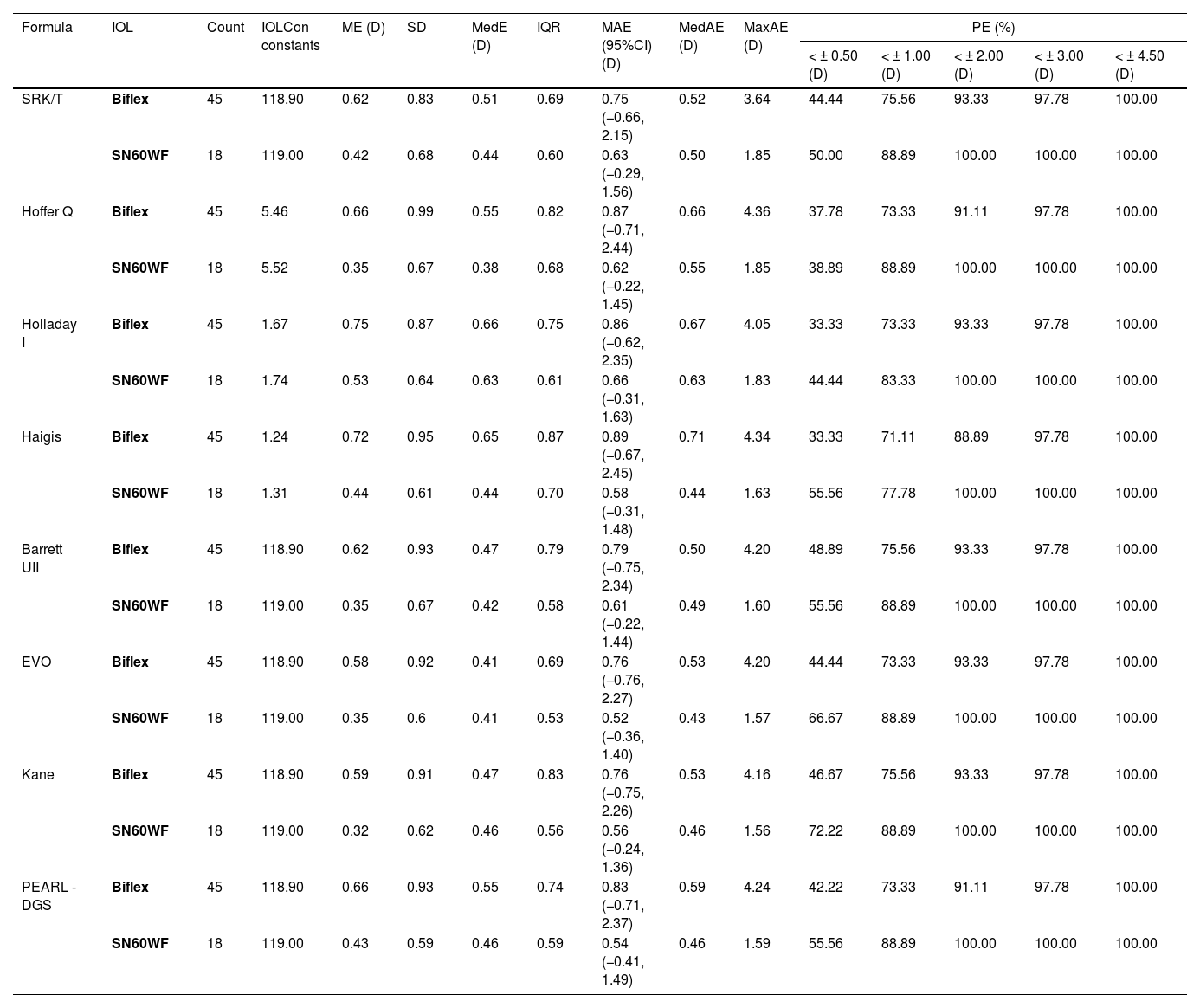
Subsequently, slight differences were observed when the constant optimization was performed for the same eyes as before. The MAE was reduced (0.65 D (−0.59, 1.88)), but the differences remained consistent across the different formulas. After constant optimization, the lowest MAE was obtained by the SRK/T (0.60 D (−0.53, 1.74)) and the highest MAE by the Hoffer Q and Haigis (0.70 D (−0.62, 2.03) and 0.69 (−0.58, 1.96), respectively) (p = 0.005). For a detailed breakdown based on the IOL model, please refer to table 3. If we analyze only the eyes in the measured group labeled as successful by the biometer, the formula with the best MAE value was SRK/T (0.32 D (−0.33, 0.98)), while the worst value was yielded by the Hoffer Q (0.42 D (−0.42, 1.26)).
Prediction error analysis for Biflex and SN60WF lens models for different formulae using optimized constants.ff Barrrett Universal II (Barrett UII), Emmetropia Verifying Optical (EVO), Intraocular Lens (IOL), Mean Error (ME), Diopters (D), Standard Deviation (SD), Median Error (MedE), Interquartile Range (IQR), Mean Absolute Error (MAE), 95% Confidence Interval (95% CI), Median Absolute Error (MedAE), Maximum Absolute Error (MaxAE), Prediction Error (PE).
The MAE differences considering all formulae with initial constants versus optimised constants were significant (p < 0.001).
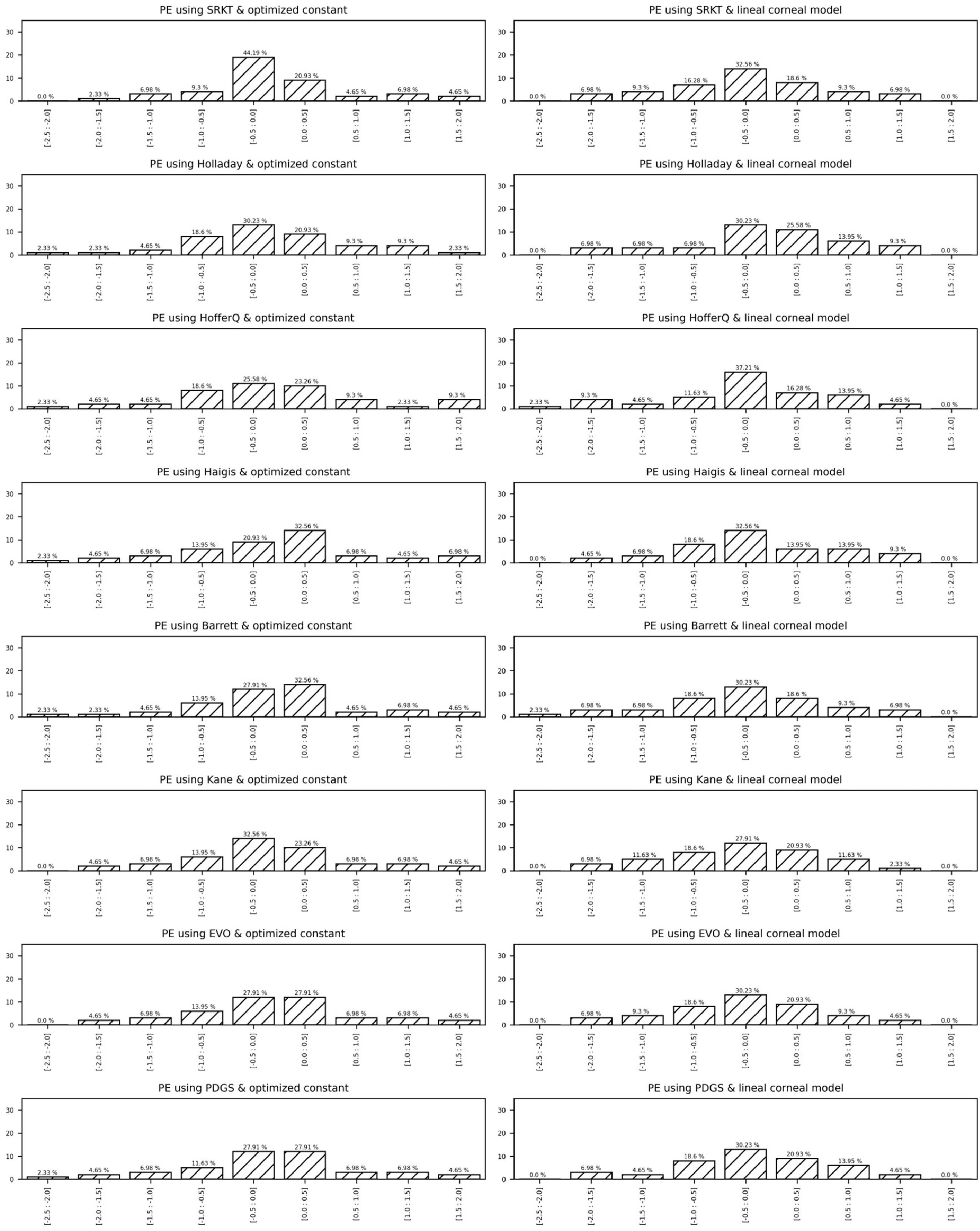
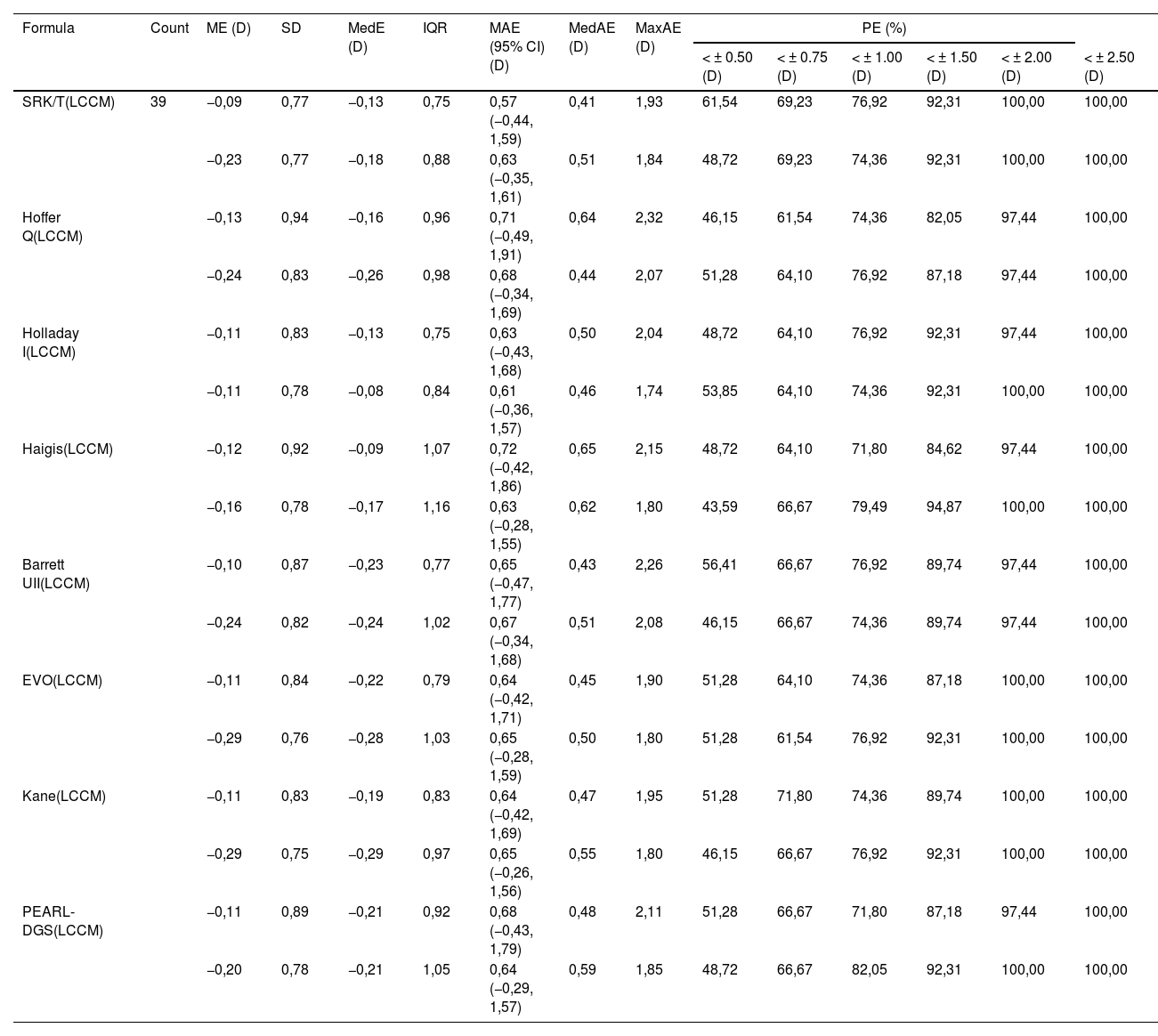
IOL calculation considering corneal changeFinally, regarding the LCCM applied to IOL calculation, only Bi-Flex IOLs with preoperative CR data available were considered (N = 39). No statistical differences were found between the optimization procedure and the performance of the LCCM analysed by formula (p = 0.38). Holladay I LCCM outperformed the other formulas in terms of MAE with 0.61 D (−0.36, 1.57), while Hoffer Q LCCM showed the highest MAE at 0.68 D (−0.34, 1.69) (p = 0.53). The mean of the maximum absolute error (MaxAE) assessed with all formulas and the optimization method was 2.08 D (1.77, 2.39), while for the LCCM method was 1.87 D (1.62, 2.12). More information is available in table 4. Prediction error distribution analysed by formula and method is shown in Fig. 3. The Kane formula adjusted with LCCM showed the best results for hyperopic surprises, with only 2.33% of eyes within the 1 to 1.5 D range.
Prediction error analysis for Biflex lens models with available preoperative corneal ratio data for different formulae with optimized constants versus with IOLCon constants considering corneal changes.gg Linear Corneal Change Model (LCCM), Barrett Universal II (Barrett UII), Emmetropia Verifying Optical (EVO), Intraocular Lens (IOL), Mean Error (ME), Diopters (D), Standard Deviation (SD), Median Error (MedE), Interquartile Range (IQR), Mean Absolute Error (MAE), 95% Confidence Interval (95% CI), Median Absolute Error (MedAE), Maximum Absolute Error (MaxAE), Prediction Error (PE).
Error intervals for the different intraocular lens calculation formulas after constant optimization and considering corneal change approaches in the subgroup of eyes with Bi-Flex intraocular lenses with successful biometric measurements of both corneal surfaces.cc Prediction error (PE).
As DMEK procedure can be performed through a 3-mm or smaller incision and the graft has a uniform thickness over its entire diameter to selectively replace the dysfunctional corneal endothelium, the procedure should induce little refractive change. However, a hyperopic shift during the postoperative period has been described due to a steepening in the posterior corneal surface as a consequence of corneal swelling resolution.8–10 There are discrepancies in the literature regarding anterior corneal surface changes following DMEK. Some authors have published series reporting insignificant changes.8,9,21 While others have published series with a significant flattening of the front surface, as in our sample.22–24 A recent published study has reported that DMEK-induced refractive shift (DIRS) and DMEK-induced IOL calculation error (DICE) were mainly associated with variations in anterior average radii of curvature (ARC), posterior average radii of curvature (PRC), PRC/ARC ratio and posterior corneal surface asphericity.25 In this study, we analyzed corneal power changes using TK, which considers both the anterior and posterior corneal surfaces. Additionally, we included SimK, which assumes a fictitious refractive index of 1.3375, as it remains a widely used clinical practice. However, we acknowledge that this assumption may introduce measurement biases. Furthermore, the exact mathematical algorithm used by the IOL Master 700 to compute TK values is not disclosed, which represents a limitation in fully understanding the underlying calculations. On the other hand, there is greater consensus among studies regarding the time when refraction stabilizes after DMEK, which typically occurs around the third month after surgery.8,24,26
As described in the literature and supported by our findings, obtaining accurate keratometric measurements in patients with FECD may be more challenging.27 Nevertheless, persevering in obtaining measurements labelled as “Successful” by the biometer would be advantageous, as this approach would lead to a lower dispersion of data and enhance the predictability of refractive outcomes. We have shown a reduction in centroid ellipsoid radii of the DAVDs when analysing the change in astigmatism in this subgroup (Fig. 2). Furthermore, we have noticed a notable decrease in MaxAE values when conducting the analysis on those eyes with available preoperative corneal ratio data (table 4) compared to those obtained in the subgroup of eyes whose only premise was that they had keratometric biometric inputs (table 3). The 9.3% of the eyes in our sample could not be fully measured with the IOL Master 700 biometer, requiring manual entry of keratometric measurements obtained from the autorefractometer for IOL assessments. This practice was associated with higher MAE values compared to eyes with successful biometry measurements, suggesting a potential source of bias. Therefore, whenever possible, efforts should be made to improve corneal oedema and surface before attempting biometry.
In line with the previous, it had been published that using adjusted conventional keratometry based on the postoperative posterior to preoperative anterior corneal curvature radii (PPPA) ratio and a fictious refractive index (FRI), reduces hyperopic DIRS, thus providing more accurate refractive outcomes than using standard keratometry; and it is no less effective than selecting a myopic target between −0.50 and −1.00 D28 On the other hand, a study on eyes undergoing triple-DMEK has been published more recently in which the authors concluded that the prediction accuracy of standard keratometry was superior to TK, being the SRK/T and multivariate formulas with the IOLup1D adjustment the most accurate.27 These findings are consistent with the results of our study. Even though the SRK/T formula with optimized constant was the one which showed a better performance (table 4 and Fig. 3), the differences between formulas for a given method is small. Other study reported that the Haigis formula was the most accurate, but our results did not identify it as superior, but also found it to be among the formulas with the worst MAE results across all groups, further supporting the variability in formula performance depending on the study population and methodology.29
With respect to our adjustment method, even though linear regression was chosen based on the observed linear trend in Fig. 1 and its simplicity for clinical application, we acknowledge its limitations, including the potential presence of non-linear relationships. To validate our approach, we conducted additional statistical analyses with Shapiro-Wilk test, confirming that both the response and explanatory variables follow a normal distribution (p = 0.41). Additionally, Pearson's correlation coefficient (r = 0.62) indicates a moderate-to-strong correlation. These results provide further support for the appropriateness of the linear regression model in this context. Although the MAE values were generally slightly higher in the LCCM version of the formulas as we used the initial constants, the distribution of the percentage of eyes within the error intervals was comparable to the formulas with optimized constants (table 4 and Fig. 3) and, of course, superior to the formulas with the initial constants (table 2). Although our conclusions are primarily based on MAE, as suggested by best practice guidelines, other authors also highlight the relevance of the median absolute error and interquartile range as complementary metrics for assessing prediction errors.16,30 In this regard, we observe a similar trend in our tables, further supporting the robustness of our findings.
The benefit of developing methods that compensate for refractive errors such as constant optimization or the LCCM that we have presented, is that in these cases the surgeon will aim for a biometric target of around 0, without having to make a personal compensation by aiming at a range without having a specific target.
Historically, FECD eyes that underwent cataract surgery were only candidates for monofocal IOL implantation, as premium IOLs were considered a relative contraindication.31 However, recent studies have suggested that premium IOL implantation can be safe and effective in selected FECD patients, following successful DMEK surgery and performing biometry once postoperative stability has been achieved.32 In these cases, highly accurate IOL power calculations are essential to avoid postoperative residual refractive errors that may lead to patient dissatisfaction.33 Our IOL calculation method could be a valuable tool to achieve such precision if trends continue along these lines.
To our knowledge there are scarce published studies on IOL constant optimization in patients with FECD, and few have evaluated the influence of considering corneal changes in the IOL power calculation as we did. Among the benefits of the innovative LCCM method over the traditional optimization IOL method is that ours streamlines the IOL selection process, as it could be implemented with a simple formula, while the other requires large collection of data. Additionally, the selection of the most appropriate IOL power based on LCCM is conceptually different from the optimization method. In our approach, the most suitable IOL for a specific eye is proposed individually through the estimation of the postoperative corneal refractive change. Furthermore, the ability of the LCCM to reduce extreme refractive surprises may have significant clinical implications, improving both surgical predictability and patient satisfaction. Limitations of our study are related to the inclusion of patients with different degrees of FECD and cataract, which may introduce heterogeneity into the results. Although the surgical technique was consistent, surgeries were performed by three different surgeons, which could introduce inter-surgeon variability. Additionally, both eyes of the same patient were analysed in some cases to ensure a sufficiently large sample size, potentially introducing clustering effects. Moreover, the monocentric design and relatively short follow-up period may limit the generalizability of our findings. Finally, our results may also not be applicable in eyes with extreme AL and Km values.
In conclusion, the anterior corneal surface has a non-negligible influence on the refractive changes that occur after Triple-DMEK surgery. Obtaining accurate biometric measurements in patients with FECD is highly recommended. It is advisable to perform IOL constant optimization to address hyperopic DIRS. Formulas adjusted with the LCCM yield comparable refractive results to those obtained with the traditional constant optimization method. Additional prospective multicentre studies are warranted to delve deeper into these analyses and further investigate the implications and generalizability of our findings. Specifically, multicenter validation is crucial for assessing the robustness of our results across diverse clinical settings. Variability in surgical techniques, biometry devices and patient demographics, which are common across different centers, could significantly influence outcomes. By including a wider array of practices and populations, we can ensure the reliability and applicability of these findings in various real-world contexts. Such studies would not only enhance the generalizability of our conclusions but also provide valuable insights into IOL selection approaches for broader patient groups.
Authors’ contributionsConceived and designed the analysis: NLB, GVR, BGS, IJA and NAA.
· Collected the data: NLB and SCA.
· Performed the analysis: GVR.
· Wrote the paper: NLB, GVR and NAA.
Data accessibility statementThe data that support the findings of this study are available from the corresponding author, Gonzalo Velarde-Rodríguez, upon reasonable request.
FundingThis research did not receive any specific grant from funding agencies in the public, commercial, or not-for-profit sectors.
Meeting presentation: Partial results of this work were presented before the 14TH EuCornea Congress on May 26–28, 2023 (Barcelona) as a poster.
Total Keratometry (TK), Simulated Keratometry (SimK).
Simulated Keratometry (SimK), Total Keratometry (TK), Against-The-Rule astigmatism (ATR), With-The-Rule astigmatism (WTR), Oblique astigmatism (OBL), Diopters (D).
Prediction error (PE).
Simulated Keratometry (SimK), Diopters (D), Mean corneal power (Km), Anterior Chamber Depth (ACD), Millimeters (mm), Axial Length (AL), Lens Thickness (LT), Central Corneal Thickness (CCT), White-to-White corneal diameter (WW), Standard Deviation (SD), Minimum value (Min), Interquartile Range (IQR), Maximum value (Max).
Barrett Universal II (Barrett UII), Emmetropia Verifying Optical (EVO), Intraocular Lens (IOL), Mean Error (ME), Diopters (D), Standard Deviation (SD), Median Error (MedE), Interquartile Range (IQR), Mean Absolute Error (MAE), 95% Confidence Interval (95% CI), Median Absolute Error (MedAE), Maximum Absolute Error (MaxAE), Prediction Error (PE).
Barrrett Universal II (Barrett UII), Emmetropia Verifying Optical (EVO), Intraocular Lens (IOL), Mean Error (ME), Diopters (D), Standard Deviation (SD), Median Error (MedE), Interquartile Range (IQR), Mean Absolute Error (MAE), 95% Confidence Interval (95% CI), Median Absolute Error (MedAE), Maximum Absolute Error (MaxAE), Prediction Error (PE).
Linear Corneal Change Model (LCCM), Barrett Universal II (Barrett UII), Emmetropia Verifying Optical (EVO), Intraocular Lens (IOL), Mean Error (ME), Diopters (D), Standard Deviation (SD), Median Error (MedE), Interquartile Range (IQR), Mean Absolute Error (MAE), 95% Confidence Interval (95% CI), Median Absolute Error (MedAE), Maximum Absolute Error (MaxAE), Prediction Error (PE).
- Home
- All contents
- Publish your article
- About the journal
- Metrics
- Download PDF
- Bibliography
- Additional material